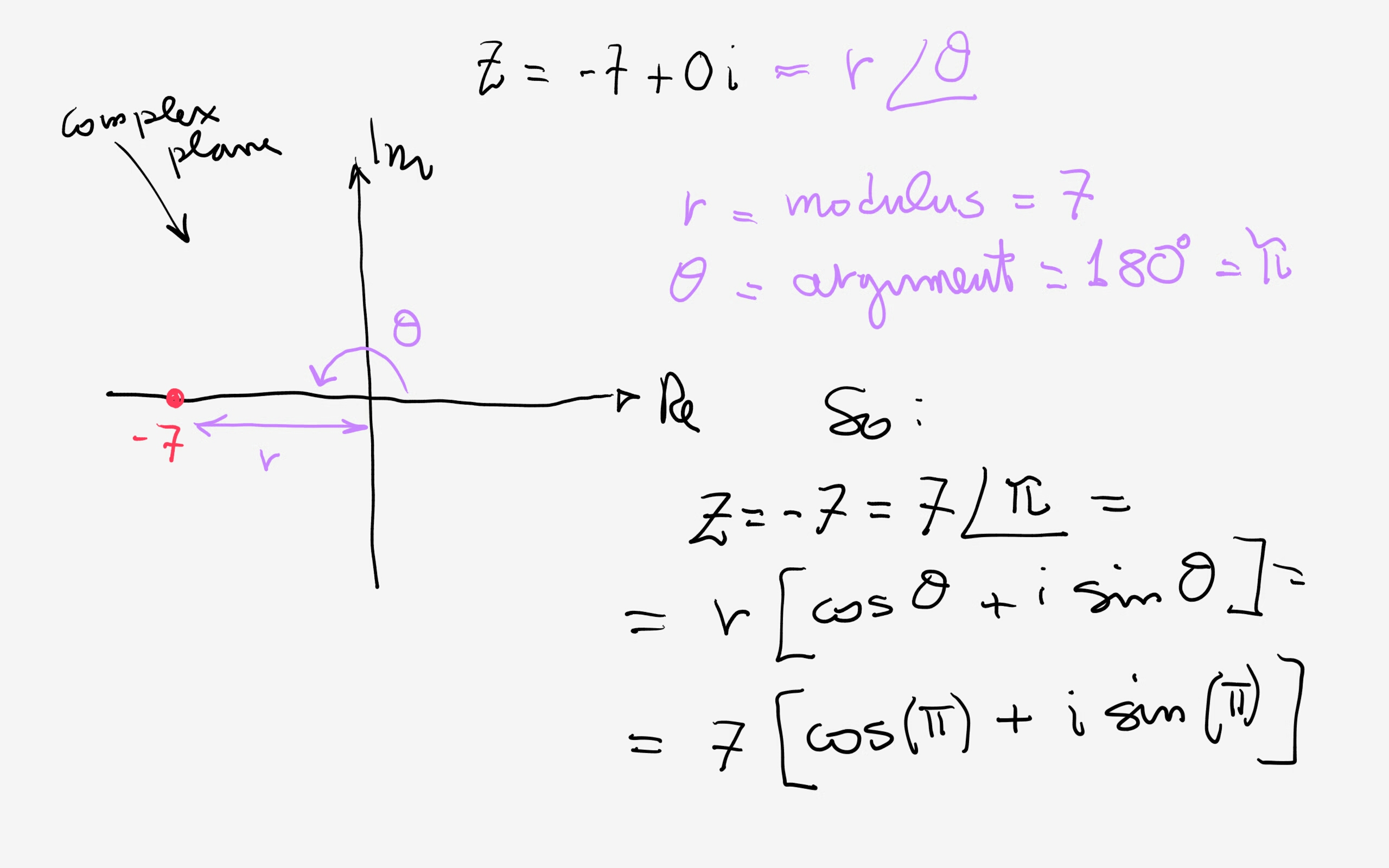Complex Numbers To Trig Form
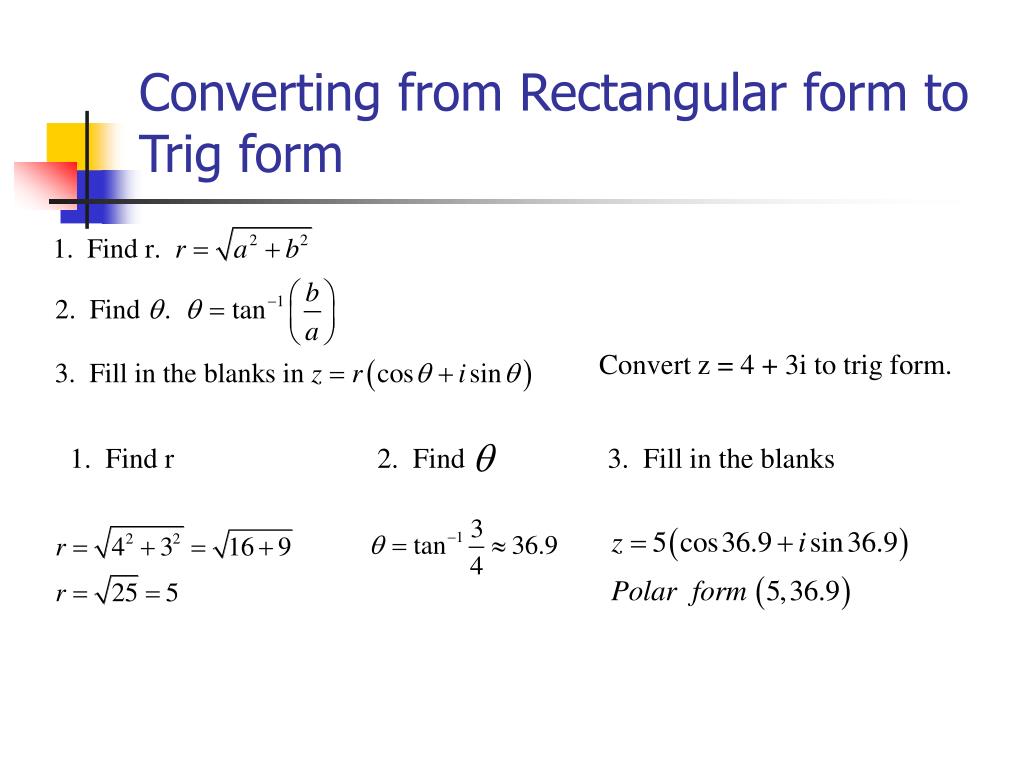
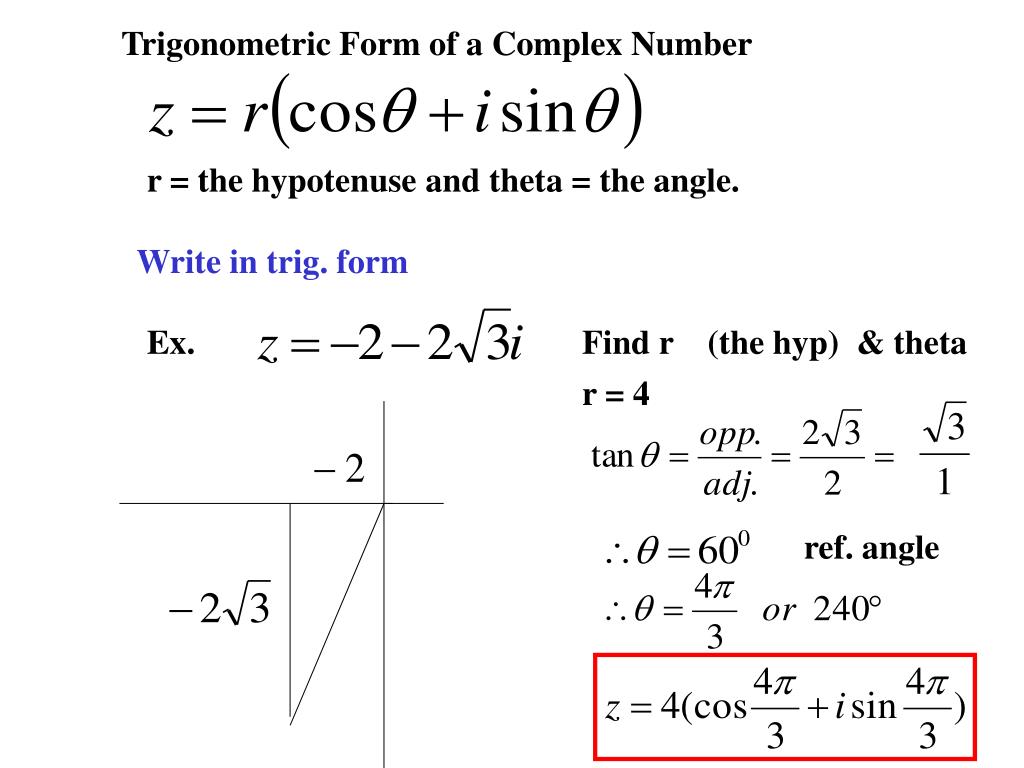
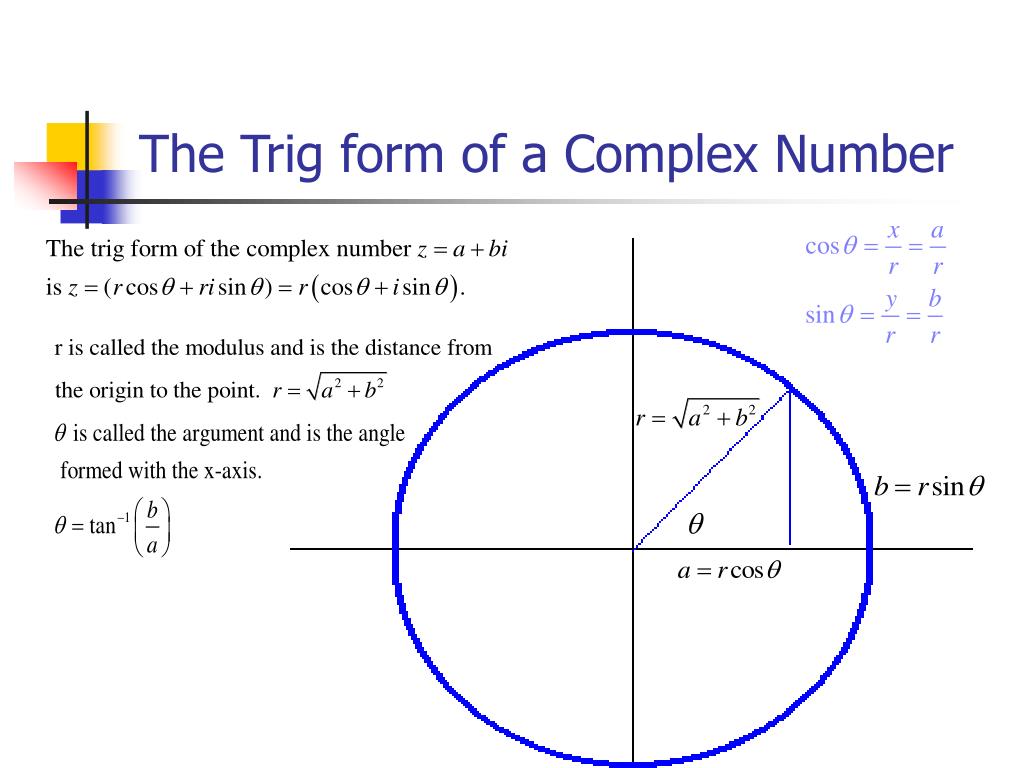
Complex Numbers To Trig Form - Z = a + b i = r ( cos θ + i sin θ), where we usually require that 0 ≤ θ ≤ 2 π. Note that z ¯ z + z + z ¯ + 1 ∈ r after this step you still should choose another representation for z. Where r = ja + bij is the modulus of z, and tan we will require 0 < 2. Click the blue arrow to submit. The modulus of a complex number is the distance from the origin on the. While rectangular form makes addition/subtraction of complex numbers easier to conceive of, trigonometric form is the best method of conceiving of complex for multiplication/division purposes. For example, you can convert complex number from algebraic to trigonometric representation form or from. $z = r (\cos \alpha + i\cdot \sin \alpha ),$ where $\alpha \in\mbox {arg} (z).$ $r,$ the modulus, or the absolute value. A complex number written as r ( cos θ + i sin θ) is said to be in trigonometric form. This is called the trigonometric form or polar form.
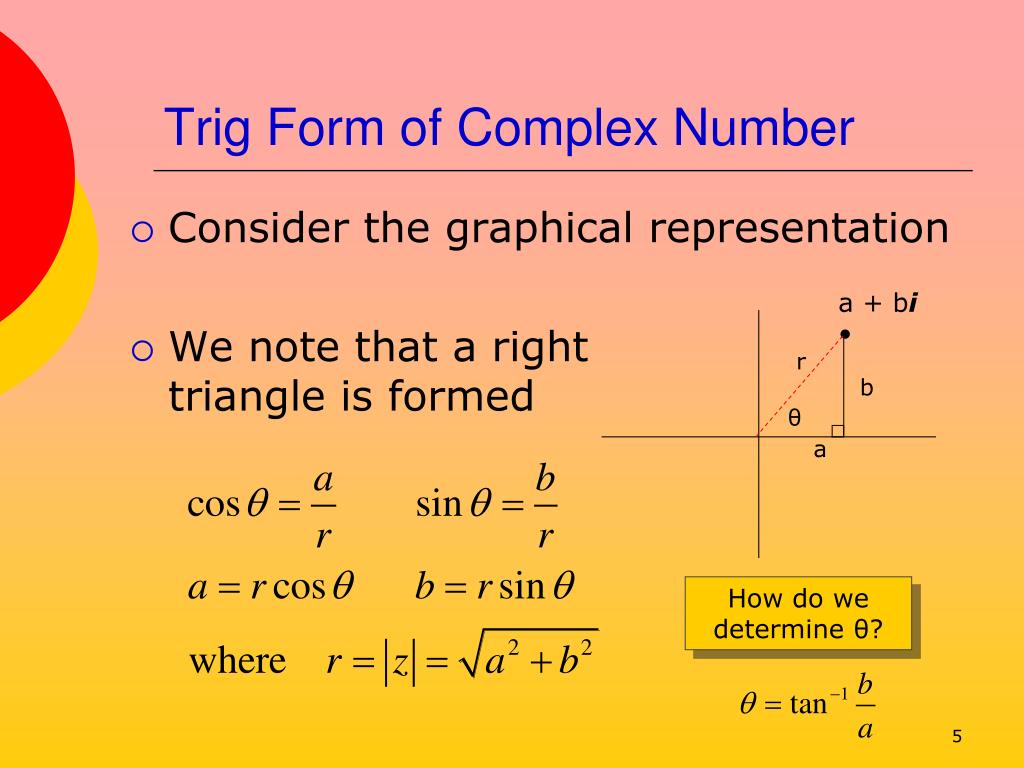
This is called the complex number’s absolute value or its modulus. Web to better understand the product of complex numbers, we first investigate the trigonometric (or polar) form of a complex number. This is called the trigonometric form or polar form. While rectangular form makes addition/subtraction of complex numbers easier to conceive of, trigonometric form is the best method of conceiving of complex for multiplication/division purposes. $$z = r\left (\cos θ + i \sin θ\right)$$. Web any complex number z = x + iy, and its complex conjugate, z = x − iy, can be written as where x = re z is the real part, y = im z is the imaginary part, r = |z| = √x2 + y2 is the magnitude of z and φ = arg z = atan2 (y, x). Web multiplying and dividing two complex numbers in trigonometric form: Web from the graph, a = cos θ and b = r sin θ. Web to find the nth root of a complex number in polar form, we use the n th n th root theorem or de moivre’s theorem and raise the complex number to a power with a rational exponent. This trigonometric form connects algebra to trigonometry and will be useful for quickly and easily finding powers and roots of complex.
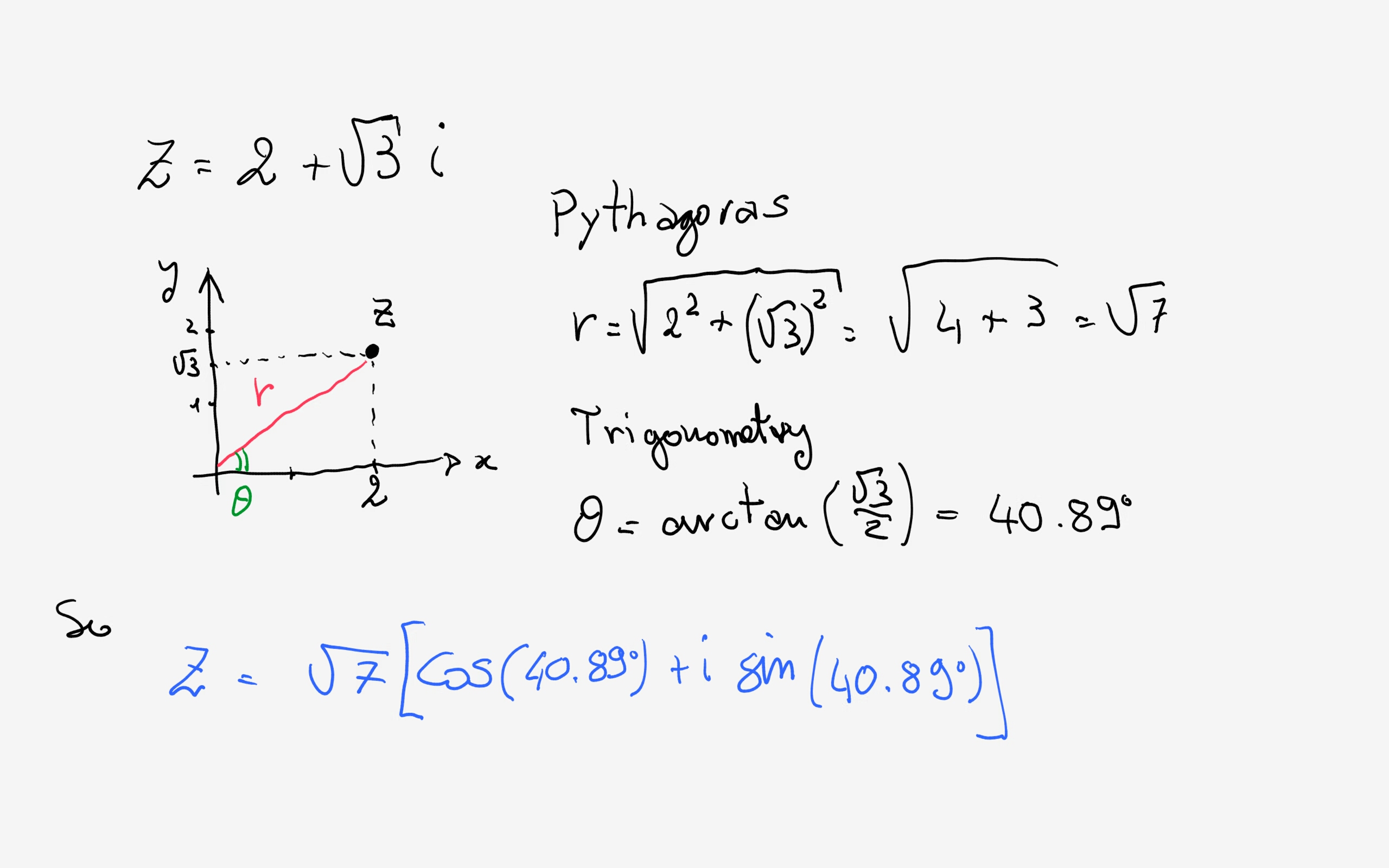
A complex number is a number that can be expressed in the form a + bi, where a and b are real numbers and i is the imaginary. Also from the graph \ (r = \sqrt {a^2 + b^2}\) and \ (\tan θ = \frac {b} {a}\). $$z = a + bi$$. Web the trigonometric form of a complex number z = a + bi is = r(cos i sin ); A complex number written as r ( cos θ + i sin θ) is said to be in trigonometric form. By nature of complex numbers, this. Reorder 5i 5 i and 3 3. This is the trigonometric form of a complex number where |z| | z | is the modulus and θ θ is the angle created on the. Web trigonometric form of complex numbers except for $0,$ any complex number can be represented in the trigonometric form or in polar coordinates: The modulus of a complex number is the distance from the origin on the.
PPT Trigonometric Form of a Complex Number PowerPoint Presentation
4 + 4i to write. Web any complex number z = x + iy, and its complex conjugate, z = x − iy, can be written as where x = re z is the real part, y = im z is the imaginary part, r = |z| = √x2 + y2 is the magnitude of z and φ = arg.
How do you express the complex number in trigonometric form 2+(sqrt 3
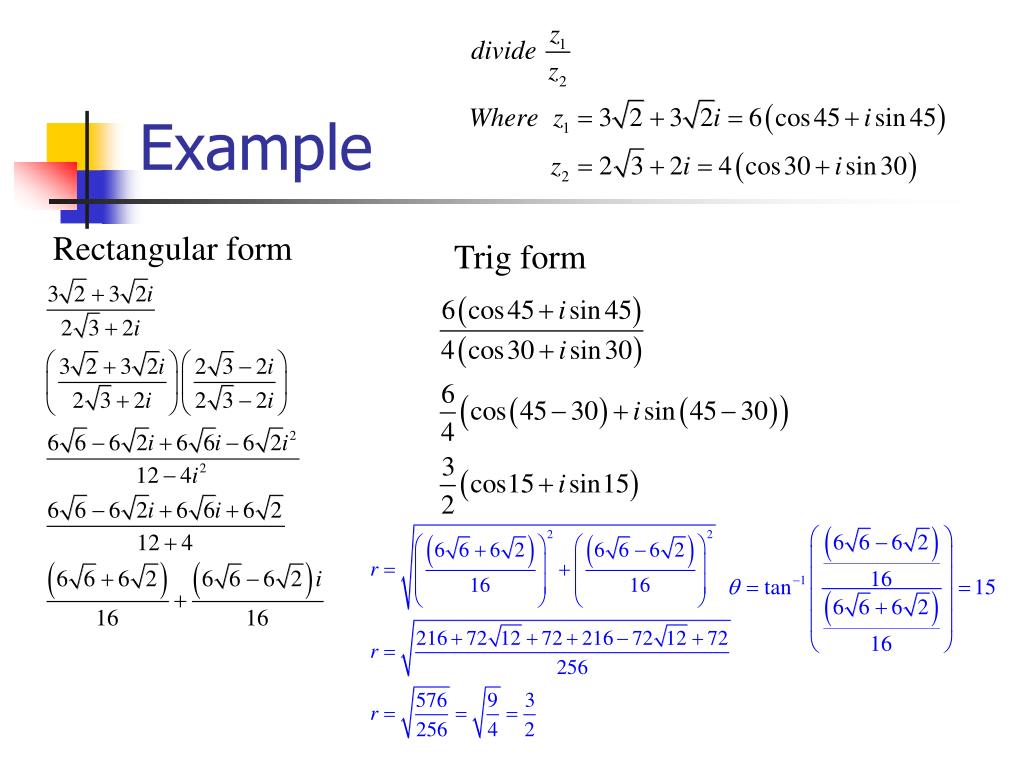
There are several ways to represent a formula for finding n th n th roots of. Web multiplying and dividing two complex numbers in trigonometric form: Web multiplication of complex numbers in trig. Answered oct 15, 2014 at 21:58. If you intend to multiply two complex numbers, z1 = r1.
PPT Trigonometric Form of Complex Numbers PowerPoint Presentation
Web trigonometric form of a complex number: Web we can write the complex number in trigonometric form as follows: $z = r (\cos \alpha + i\cdot \sin \alpha ),$ where $\alpha \in\mbox {arg} (z).$ $r,$ the modulus, or the absolute value. This calculator allows one to convert complex number from one representation form to another with step by step solution..
Trigonometric Form Into A Complex Number
Reorder 5i 5 i and 3 3. Web multiplying and dividing two complex numbers in trigonometric form: Web complex number form converter. Web trigonometric form of complex numbers. If you intend to multiply two complex numbers, z1 = r1.
PPT Trigonometric Form of a Complex Number PowerPoint Presentation
Despite their names, complex numbers and imaginary numbers have very real and significant. Web trigonometric form of complex numbers except for $0,$ any complex number can be represented in the trigonometric form or in polar coordinates: Note that z ¯ z + z + z ¯ + 1 ∈ r after this step you still should choose another representation for.
The Product and Quotient of Complex Numbers in Trigonometric Form YouTube
(r cis q) (s cis j) = rs cis ( q + j ) reciprocal of complex numbers in trig. A complex number written as r ( cos θ + i sin θ) is said to be in trigonometric form. Web from the graph, a = cos θ and b = r sin θ. By nature of complex numbers, this..
18+ trigonometric form of a vector KhailaMillen
This is the trigonometric form of a complex number where |z| | z | is the modulus and θ θ is the angle created on the. $$z = r\left (\cos θ + i \sin θ\right)$$. Web multiplying and dividing two complex numbers in trigonometric form: = b is called the argument of z. For example, you can convert complex number.
Complex Numbers in Trigonometric Form YouTube
For example, you can convert complex number from algebraic to trigonometric representation form or from. Web we can write the complex number in trigonometric form as follows: Web any complex number z = x + iy, and its complex conjugate, z = x − iy, can be written as where x = re z is the real part, y =.
PPT Trigonometric Form of a Complex Number PowerPoint Presentation
By nature of complex numbers, this. Web from the graph, a = cos θ and b = r sin θ. ( r 1 ( cos ( θ 1) + i. Web how to write complex numbers in trigonometric form? Web any complex number z = x + iy, and its complex conjugate, z = x − iy, can be written.
Trig Form of Complex Numbers Part 2 VIDEO 2 YouTube
Web any complex number z = x + iy, and its complex conjugate, z = x − iy, can be written as where x = re z is the real part, y = im z is the imaginary part, r = |z| = √x2 + y2 is the magnitude of z and φ = arg z = atan2 (y, x)..
A Number In The Form A + B I, Where A And B Are Real Numbers, And I Is The Imaginary Unit, Or − 1, Is Called A Complex Number.
Note that z ¯ z + z + z ¯ + 1 ∈ r after this step you still should choose another representation for z. By nature of complex numbers, this. Web we can write the complex number in trigonometric form as follows: Also from the graph \ (r = \sqrt {a^2 + b^2}\) and \ (\tan θ = \frac {b} {a}\).
Web Multiplying And Dividing Two Complex Numbers In Trigonometric Form:
Click the blue arrow to submit. As a refresher, the distance between the origin and the complex number is equal to $|a + bi| = \sqrt{a^2 + b^2}$. $$z = a + bi$$. Web to multiply two complex numbers z1 = a + bi and z2 = c + di, use the formula:
Web To Find The Nth Root Of A Complex Number In Polar Form, We Use The N Th N Th Root Theorem Or De Moivre’s Theorem And Raise The Complex Number To A Power With A Rational Exponent.
This is called the trigonometric form or polar form. 4 + 4i to write. This is called the complex number’s absolute value or its modulus. Web to better understand the product of complex numbers, we first investigate the trigonometric (or polar) form of a complex number.
= B Is Called The Argument Of Z.
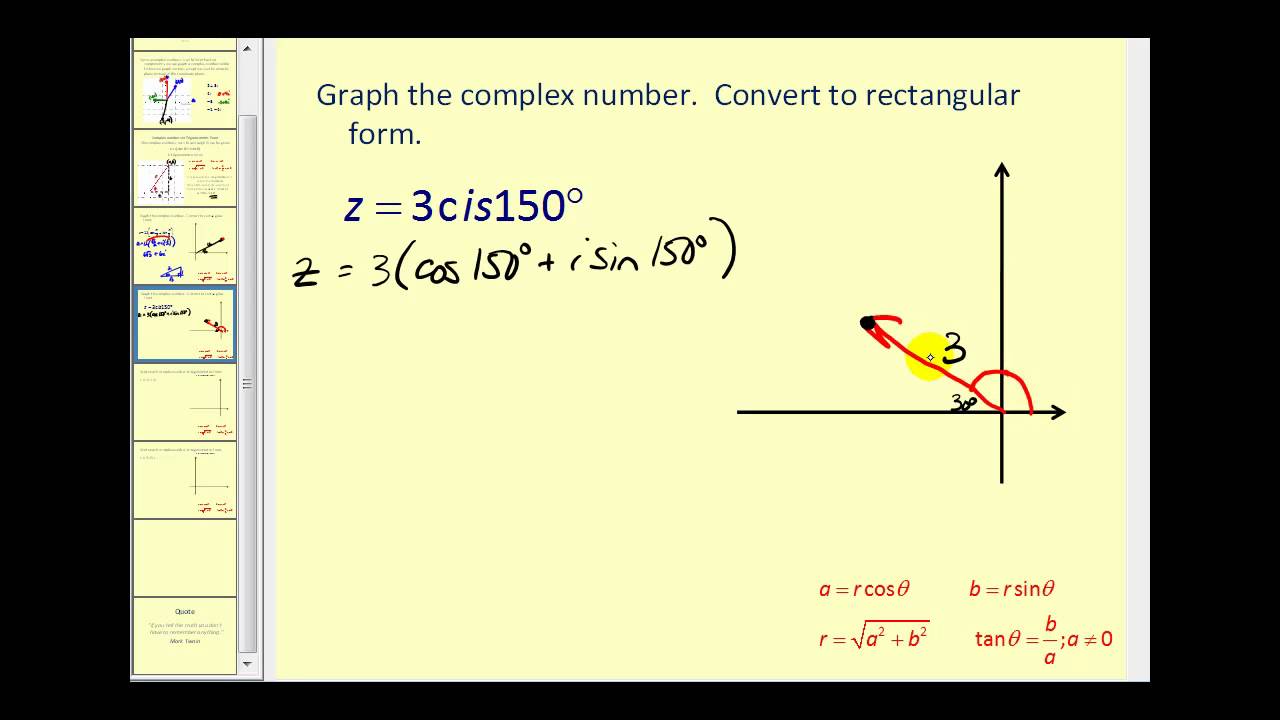
Web any complex number z = x + iy, and its complex conjugate, z = x − iy, can be written as where x = re z is the real part, y = im z is the imaginary part, r = |z| = √x2 + y2 is the magnitude of z and φ = arg z = atan2 (y, x). Web how to write complex numbers in trigonometric form? Web translate the following complex numbers from trigonometric polar form to rectangular form. The modulus of a complex number is the distance from the origin on the.