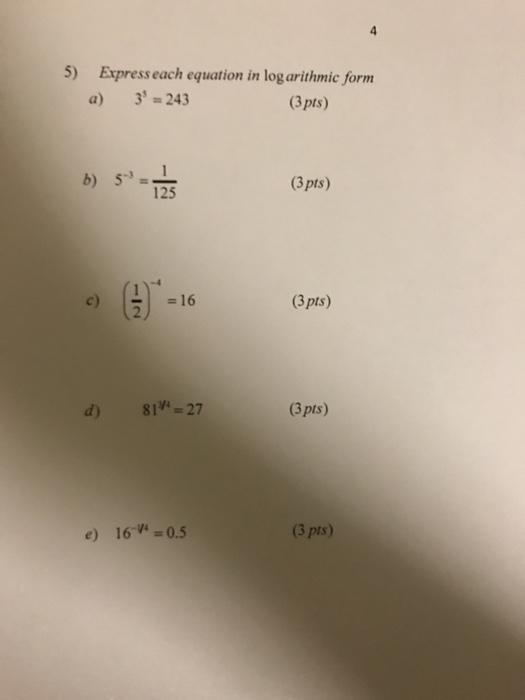Express This Equation In Logarithmic Form 23 8
Express This Equation In Logarithmic Form 23 8 - Check the full answer on app gauthmath. All logarithm solvers, trainers and word problems on this page: Web detailed steps (63) write neatly (62) help me a lot (52) easy to understand (34) correct answer (32) excellent handwriting (15) does the answer help you? Web now, we can solve for x x by converting the equation to logarithmic form. Reduce by cancelling the common factors. Convert the exponential equation to a. You'll get a detailed solution from a subject matter expert that helps you learn core concepts. (a) 23 = 8 is equivalent to the logarithmic equation: Web this problem has been solved! Web this is expressed by the exponential equation \blued2^\greene4=\goldd {16} 24 = 16.
Any exponential equation of the form ab=c can be written in logarithmic form using loga(c)=b. \blued {2}^\greend x= \goldd {48} 2x = 48 is equivalent to \log_ {\blued {2}} (\goldd. Web precalculus 8ex = 23 8 e x = 23 simplify. Web now, we can solve for x x by converting the equation to logarithmic form. Web this is expressed by the exponential equation \blued2^\greene4=\goldd {16} 24 = 16. Express this equation in logarithmic form. Enter the logarithmic expression below which you want to simplify. Ex = 23 8 e x = 23 8 convert the exponential equation to a logarithmic equation using the logarithm base (e) ( e) of. What are the 3 types of logarithms? Check the full answer on app gauthmath.
Web the 8 inside the log argument is the the exponential equation is equivalent to the log form equationthe base of the log is the same as the base of the exponent. Web logarithmic expressions and equations. The three types of logarithms. What are the 3 types of logarithms? Reduce by cancelling the common factors. Web logarithmic equations calculator online with solution and steps. Web this problem has been solved! A) 16 3/2 = 64 b) ex = 5 b. Any exponential equation of the form ab=c can be written in logarithmic form using loga(c)=b. Web express this equation in logarithmic form.
Express the equation in logarithmic form (a)
Ex = 23 e x = 23. Convert the exponential equation to a logarithmic. 25 = x2 25 = x 2. Detailed step by step solutions to your logarithmic equations problems with our math solver and online. All logarithm solvers, trainers and word problems on this page:
Solved 5) Express each equation in logarithmic form a) 243
Web logarithmic equations calculator online with solution and steps. Enter the logarithmic expression below which you want to simplify. Reduce by cancelling the common factors. Web express the following equations in logarithmic form: Any exponential equation of the form ab=c can be written in logarithmic form using loga(c)=b.
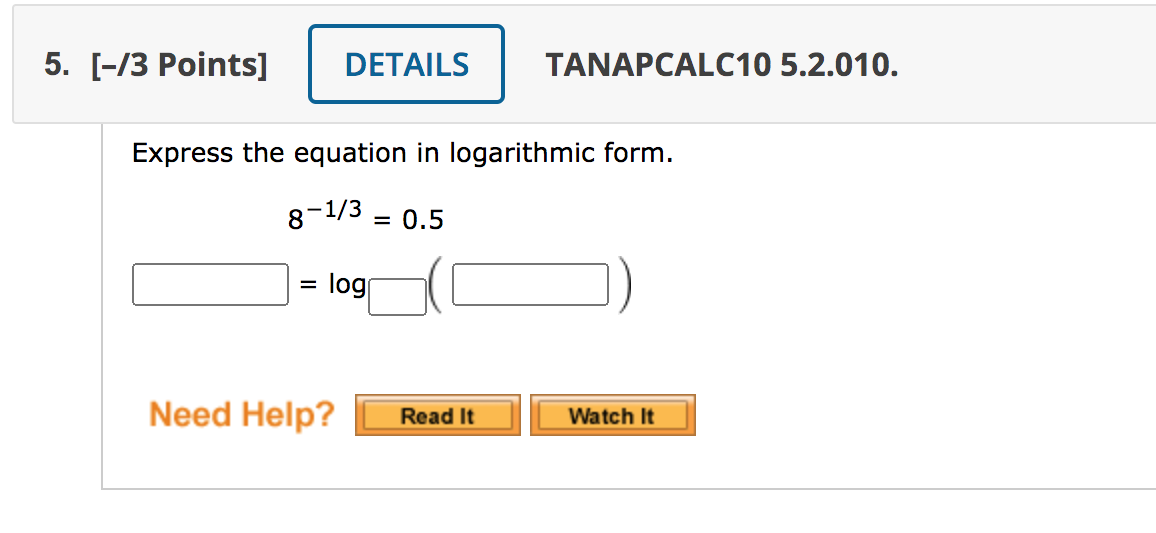
Solved Express the equation in logarithmic form.
Web answers archive back to: Web a logarithmic equation is an equation that involves the logarithm of an expression containing a varaible. Convert the exponential equation to a logarithmic. Enter the logarithmic expression below which you want to simplify. A) 16 3/2 = 64 b) ex = 5 b.
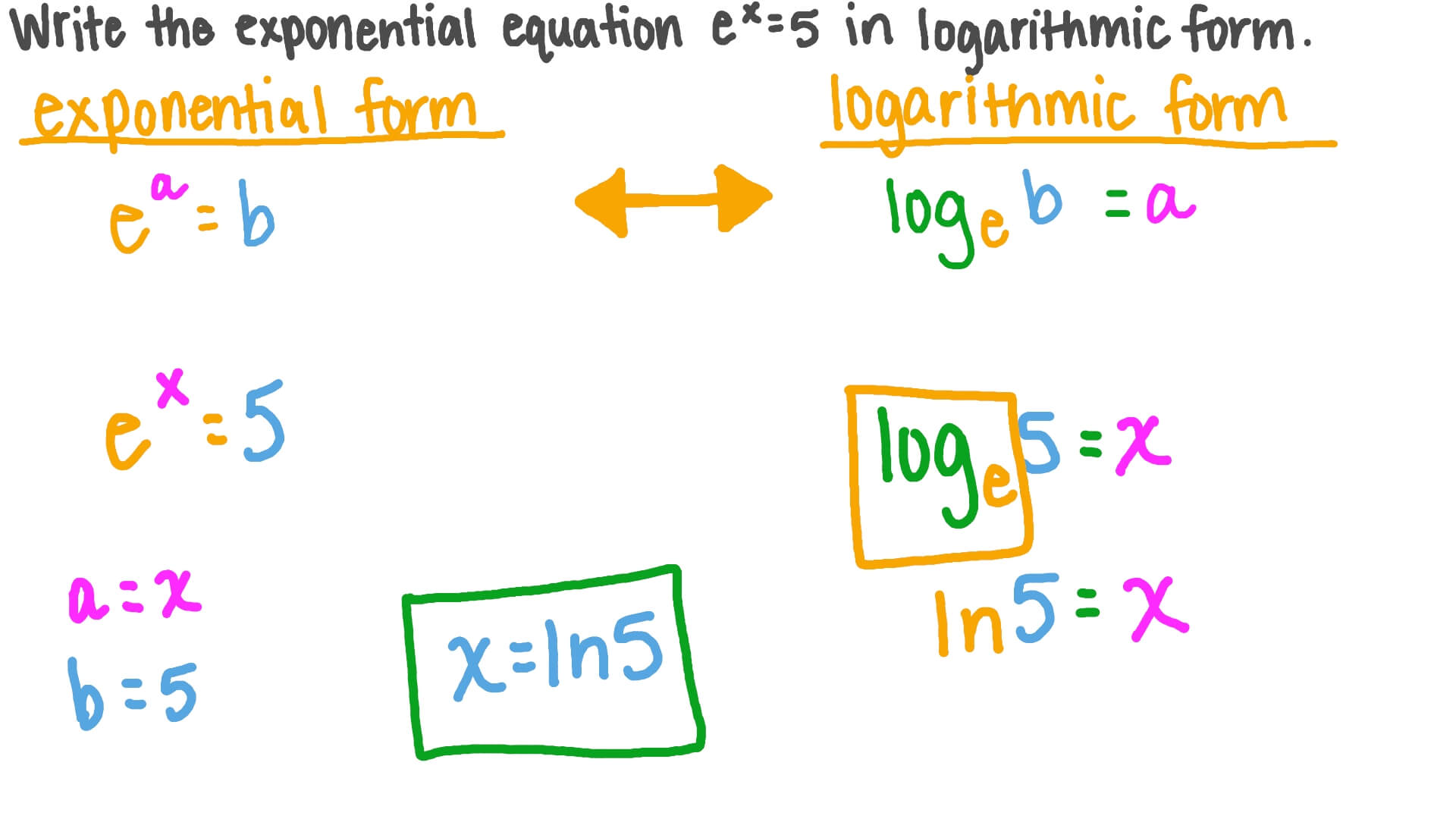
Question Video Rewriting an Exponential Equation in Logarithmic Form
Ex = 23 e x = 23. Detailed step by step solutions to your logarithmic equations problems with our math solver and online. Web this is expressed by the exponential equation \blued2^\greene4=\goldd {16} 24 = 16. Web precalculus 8ex = 23 8 e x = 23 simplify. Web now, we can solve for x x by converting the equation to.
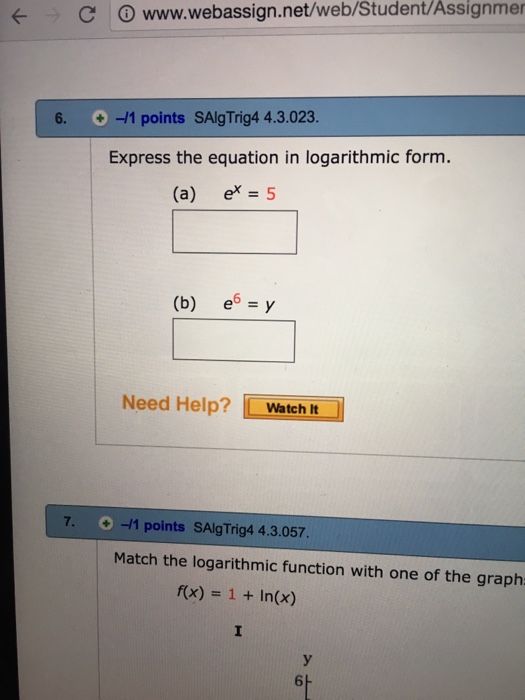
Solved Express The Equation In Logarithmic Form. (a) E^x
\blued {2}^\greend x= \goldd {48} 2x = 48 is equivalent to \log_ {\blued {2}} (\goldd. Convert to logarithmic form e^x=23. Web this is expressed by the exponential equation \blued2^\greene4=\goldd {16} 24 = 16. What are the 3 types of logarithms? 25 = x2 25 = x 2.
Answered Express the equation in logarithmic… bartleby
Ex = 23 8 e x = 23 8 convert the exponential equation to a logarithmic equation using the logarithm base (e) ( e) of. 25 = x2 25 = x 2. Ex = 23 e x = 23. Web now, we can solve for x x by converting the equation to logarithmic form. \blued {2}^\greend x= \goldd {48} 2x.
Solved Express the equation in logarithmic form (a) e 4 is
The three types of logarithms. Ex = 23 e x = 23. Reduce by cancelling the common factors. Web express the following equations in logarithmic form: 25 = x2 25 = x 2.
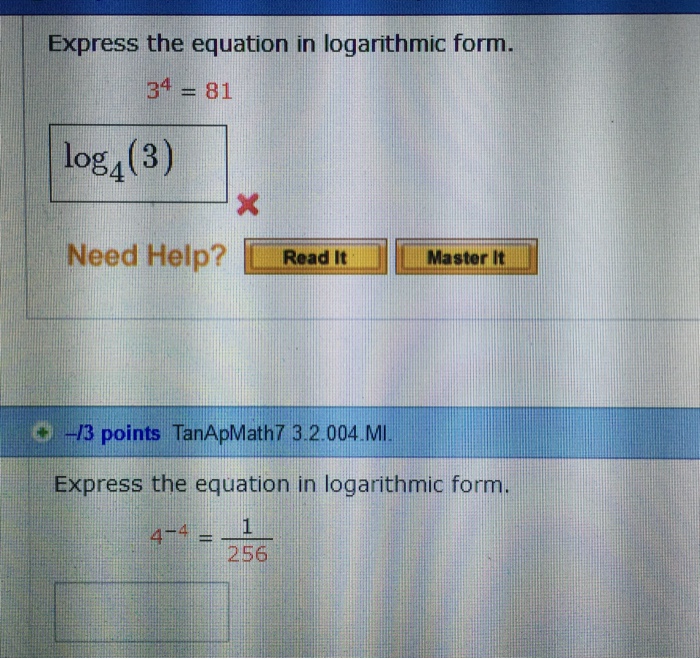
Solved Express the equation in logarithmic form. 3^4 = 81
Convert the exponential equation to a logarithmic. Any exponential equation of the form ab=c can be written in logarithmic form using loga(c)=b. Check the full answer on app gauthmath. Web which of the functions below is the inverse of the logarithm function? Ex = 23 e x = 23.
[Solved] Solve the following logarithmic equation. Express irrational
25 = x2 25 = x 2. Web answers archive back to: Ex = 23 e x = 23. Reduce by cancelling the common factors. Web which of the functions below is the inverse of the logarithm function?
Answered Express the equation in logarithmic… bartleby
25 = x2 25 = x 2. All logarithm solvers, trainers and word problems on this page: (a) 23 = 8 is equivalent to the logarithmic equation: \blued {2}^\greend x= \goldd {48} 2x = 48 is equivalent to \log_ {\blued {2}} (\goldd. Express this equation in logarithmic form.
(A) 23 = 8 Is Equivalent To The Logarithmic Equation:
What are the 3 types of logarithms? Web detailed steps (63) write neatly (62) help me a lot (52) easy to understand (34) correct answer (32) excellent handwriting (15) does the answer help you? \blued {2}^\greend x= \goldd {48} 2x = 48 is equivalent to \log_ {\blued {2}} (\goldd. Web the 8 inside the log argument is the the exponential equation is equivalent to the log form equationthe base of the log is the same as the base of the exponent.
Ex = 23 E X = 23.
Web how to convert to logarithmic form. Any exponential equation of the form ab=c can be written in logarithmic form using loga(c)=b. When you are given an equation and asked to 'express it in logarithmic form',. Web this is expressed by the exponential equation \blued2^\greene4=\goldd {16} 24 = 16.
Web Express The Following Equations In Logarithmic Form:
Enter the logarithmic expression below which you want to simplify. Web logarithmic equations calculator online with solution and steps. (b) 10−3 = 0.001 is equivalent to the logarithmic equation:. Express this equation in logarithmic form.
Ex = 23 8 E X = 23 8 Convert The Exponential Equation To A Logarithmic Equation Using The Logarithm Base (E) ( E) Of.
Web logarithmic expressions and equations. The logarithm calculator simplifies the given logarithmic expression by using the laws of logarithms. 25 = x2 25 = x 2. Convert the exponential equation to a logarithmic.