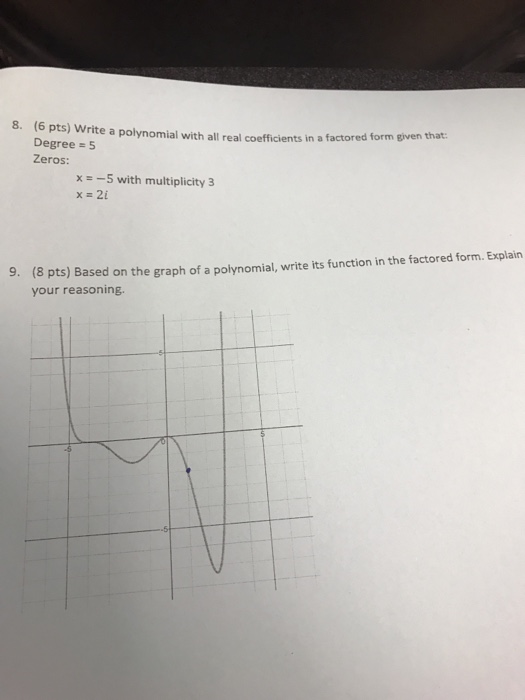
How To Write A Polynomial In Factored Form
How To Write A Polynomial In Factored Form - Web polynomial equations in factored form. F (x) = a(x−x1)p1(x−x2)p2 ⋯(x−xn)pn f ( x) = a ( x − x 1) p 1 ( x − x 2) p 2 ⋯ ( x − x n) p n where the powers pi p i on each factor can. Organize factors (left to right) from smallest zero to largest. Just like numbers have factors (2×3=6), expressions have factors ( (x+2) (x+3)=x^2+5x+6). Factoring polynomials with quadratic forms. We begin by looking at the following example: For example, the gcf of 6x 6x and 4x^2 4x2 is 2x 2x. In such cases, the polynomial is said to factor over the rationals. factoring is a useful way to find rational roots (which correspond to linear factors) and simple roots involving. Here you will learn how to solve polynomials in expanded form. Then, for example, if 2 ∈ r 2 ∈ r (this is your α.
Write each of the polynomial function in factored form. In such cases, the polynomial is said to factor over the rationals. factoring is a useful way to find rational roots (which correspond to linear factors) and simple roots involving. Here you will learn how to solve polynomials in expanded form. = 6x2 + 3x = 3x(2x + 1). Zeros of multiplicity 2 at x=3 and x=1 and a zero of multiplicity 1. Factoring polynomials with quadratic forms. Just like numbers have factors (2×3=6), expressions have factors ( (x+2) (x+3)=x^2+5x+6). When irreducible quadratic factors are set to zero and solved for x, imaginary solutions are produced. 1.8m views 3 years ago. Web yes, if α ∈ f α ∈ f, then by f(α) f ( α) we just mean the polynomial obtained by replacing each occurence of x x by α α.
\[{x^2} + 6x + 9 = \left( {x + 3} \right)\left( {x + 3} \right) = {\left( {x + 3} \right)^2}\] note as well that we further simplified the factoring to acknowledge that it is a perfect square. Just like numbers have factors (2×3=6), expressions have factors ( (x+2) (x+3)=x^2+5x+6). = 6x2 + 3x = 3x(2x + 1). What you should be familiar with before this lesson the gcf (greatest common factor) of two or more monomials is the product of all their common prime factors. $$x\cdot \left ( x+4 \right )=12$$ we multiply as usual: If each term in the polynomial shares a common factor. You should always do this when it. Web to find the factored form of a polynomial, this calculator employs the following methods: Web factoring polynomials is the reverse procedure of the multiplication of factors of polynomials. = 6 x 2 + 3 x = 3 x ( 2 x + 1) \begin {aligned}&\phantom {=}~6x^2+3x\\\\&=3x (2x+1)\\\\\end {aligned}.
Write A Polynomial With All Real Coefficients In A...
Web let's get equipped with a variety of key strategies for breaking down higher degree polynomials. Web yes, if α ∈ f α ∈ f, then by f(α) f ( α) we just mean the polynomial obtained by replacing each occurence of x x by α α. Web how do you write a factored form? These are the steps for.
Solving Polynomials in Factored Form YouTube
We begin by looking at the following example: Write each repeated factor in exponential form. You can also write them horizontally and group the like terms. Web factoring polynomials is the reverse procedure of the multiplication of factors of polynomials. = 6x2 + 3x = 3x(2x + 1).
Polynomials Factored Form to Standard Form YouTube
For example, the gcf of 6x 6x and 4x^2 4x2 is 2x 2x. You can also write them horizontally and group the like terms. Write each repeated factor in exponential form. Web let's get equipped with a variety of key strategies for breaking down higher degree polynomials. Negative common factor + grouping.
Write the polynomial in factored form. p(x)=(x+5)(x___)(x+
You should always do this when it. To subtract, reverse the sign of each term in the second polynomial and add the two polynomials.follow. Web use the description below to write the formula (in factored form) for a polynomial of least degree. This video covers common terminology like terms, degree, standard form, monomial, binomial and trinomial. Web here is the.
Writing a Polynomial in Factored Form YouTube
We used the zero product principle to solve the polynomial equation 0= −1 2y(9+y) 0 = − 1 2 y ( 9 + y) sometimes solving an equation requires the combination of. Web about press copyright contact us creators advertise developers terms privacy policy & safety how youtube works test new features nfl sunday ticket press copyright. Factoring polynomials with.
Solved In row 1, write a polynomial function in factored
Web factor the polynomial as a product of linear factors (of the form (ax + b) ), and irreducible quadratic factors (of the form (ax2 + bx + c). In such cases, the polynomial is said to factor over the rationals. factoring is a useful way to find rational roots (which correspond to linear factors) and simple roots involving. These.
52 Analyze Factored form and Write a polynomial from zeros (12/16/2013
Web factoring polynomials is the reverse procedure of the multiplication of factors of polynomials. Web we used the gcf to factor the polynomial −9 2y− 1 2y2 − 9 2 y − 1 2 y 2. Negative common factor + grouping. $$x^{2}+4x=12$$ we may also do the inverse. You can also write them horizontally and group the like terms.
Characteristics of Polynomial in Factored Form Concepts YouTube
Web learn how to factor a common factor out of a polynomial expression. The degree of the polynomial is 5. Web the number of times a given factor appears in the factored form of the equation of a polynomial is called the multiplicity. Just like numbers have factors (2×3=6), expressions have factors ( (x+2) (x+3)=x^2+5x+6). Organize factors (left to right).
Solved In row 1, write a polynomial function in factored
For example, the gcf of 6x 6x and 4x^2 4x2 is 2x 2x. Write the polynomials vertically (one below the other) such that terms are aligned. Web the number of times a given factor appears in the factored form of the equation of a polynomial is called the multiplicity. Web factoring polynomials is the reverse procedure of the multiplication of.
How To Write In Factored Form
These are the steps for this process. $$x^{2}+4x=12$$ we may also do the inverse. What you should be familiar with before this lesson the gcf (greatest common factor) of two or more monomials is the product of all their common prime factors. Write the polynomials vertically (one below the other) such that terms are aligned. Web let's get equipped with.
We Begin By Looking At The Following Example:
For example, factor 6x²+10x as 2x (3x+5). The following sections will show you how to factor different polynomial. Write the polynomials vertically (one below the other) such that terms are aligned. $$x\cdot \left ( x+4 \right )=12$$ we multiply as usual:
Web Let's Get Equipped With A Variety Of Key Strategies For Breaking Down Higher Degree Polynomials.
Web factoring out common factors. For example, the gcf of 6x 6x and 4x^2 4x2 is 2x 2x. Organize factors (left to right) from smallest zero to largest. = 6 x 2 + 3 x = 3 x ( 2 x + 1) \begin {aligned}&\phantom {=}~6x^2+3x\\\\&=3x (2x+1)\\\\\end {aligned}.
The Terms Could Be Constant Or Linear Or Any Polynomial Form Which Is Not Further Divisible.
This video covers common terminology like terms, degree, standard form, monomial, binomial and trinomial. F (x) = a(x−x1)p1(x−x2)p2 ⋯(x−xn)pn f ( x) = a ( x − x 1) p 1 ( x − x 2) p 2 ⋯ ( x − x n) p n where the powers pi p i on each factor can. Web use the description below to write the formula (in factored form) for a polynomial of least degree. In such cases, the polynomial is said to factor over the rationals. factoring is a useful way to find rational roots (which correspond to linear factors) and simple roots involving.
= X 2 + 7 X + 12 = (.
Factoring gcf, 2 factoring by grouping, 3 using the difference of squares, and 4 factoring quadratic polynomials If a polynomial of lowest degree p has zeros at x= x1,x2,…,xn x = x 1, x 2,., x n , then the polynomial can be written in the factored form: = 6x2 + 3x = 3x(2x + 1). The zero associated with this factor, x = 2, x = 2, has multiplicity 2 because the factor (x − 2) (x − 2) occurs twice.