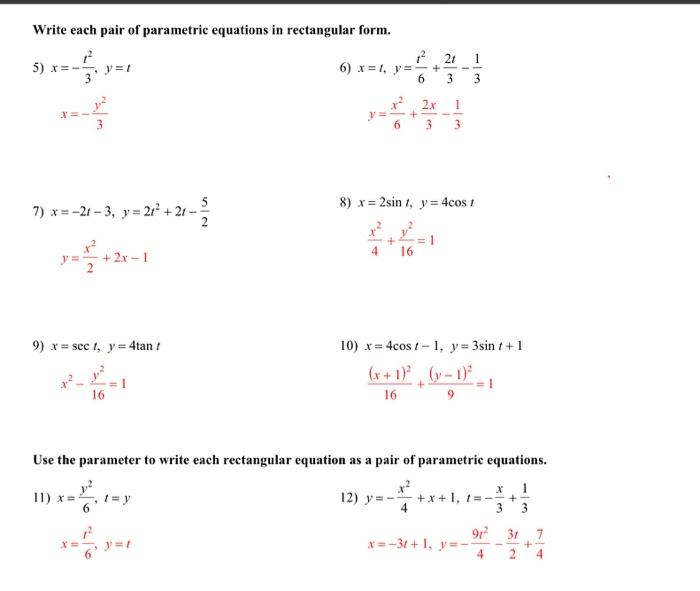
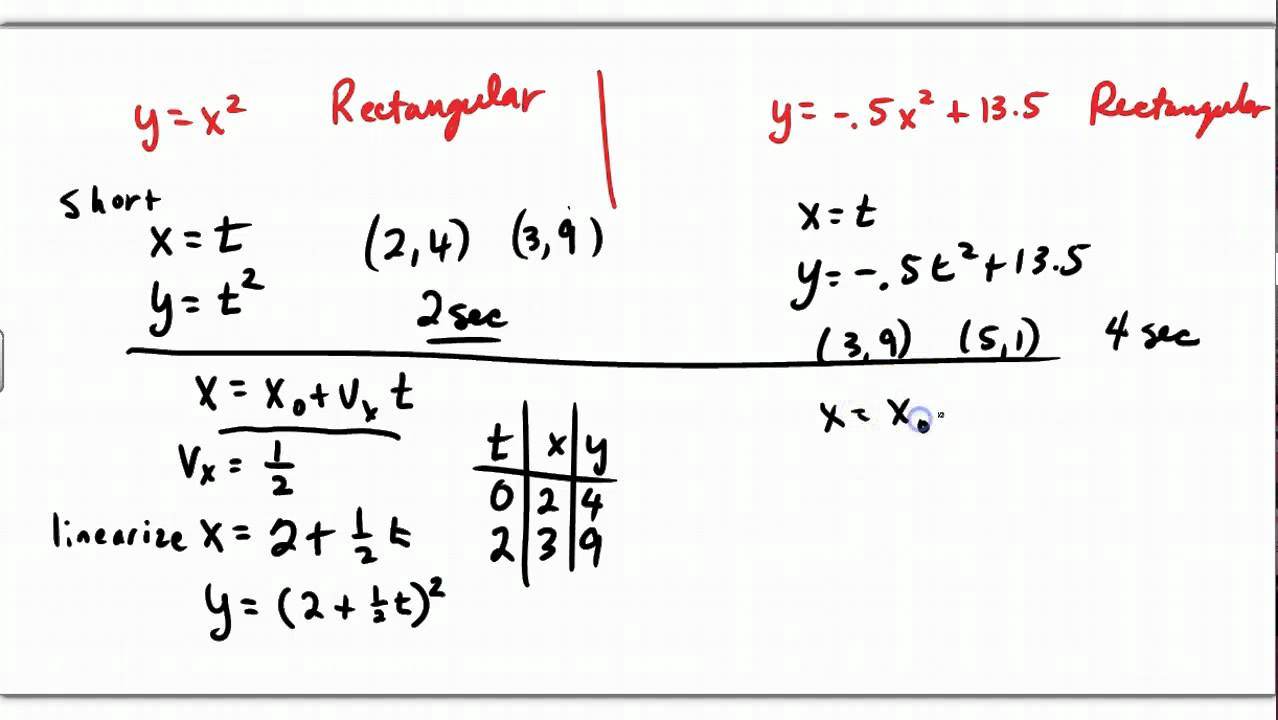
Parametric Equations In Rectangular Form
Parametric Equations In Rectangular Form - Web form a parametric representation of the unit circle, where t is the parameter: Following steps must be followed in order to convert the equation in parametric form. In this section, we consider sets of equations given by the functions x(t) and y(t), where t is the independent variable of time. X = t2 x = t 2. Web convert the parametric equations 𝑥 equals 𝑡 squared plus two and 𝑦 equals three 𝑡 minus one to rectangular form. Although we have just shown that there is only one way to interpret a set of parametric equations as a rectangular equation, there are multiple ways to interpret a rectangular equation as a set of parametric equations. You have to solve for \(t\) in one of the equations. Web convert the parametric equations 𝑥 is equal to the cos of 𝑡 and 𝑦 is equal to the sin of 𝑡 to rectangular form. A point ( x, y) is on the unit circle if and only if there is a value of t such that these two equations generate that point. Y = x 4 + 5x 2 +8;
Let’s evaluate the equation 1: Although we have just shown that there is only one way to interpret a set of parametric equations as a rectangular equation, there are multiple ways to interpret a rectangular equation as a set of parametric equations. Web write down the following mentioned rectangular equations into parametric form. 4.2k views 2 years ago parametric equations. X = t2 x = t 2 , y = t9 y = t 9. Y = 3x3 + 5x +6. By convention, t is frequently used as the parameter, though other variables can be used as well. Web form a parametric representation of the unit circle, where t is the parameter: Web a typical parametric equation will be in the form x = f ( t) and y = g ( t). For parametric equations, put x = t so, the equation becomes, y = 3t 3 + 5t + 6
Web finding parametric equations for curves defined by rectangular equations. Web write down the following mentioned rectangular equations into parametric form. Web a typical parametric equation will be in the form x = f ( t) and y = g ( t). From the curve’s vertex at (1, 2), the graph sweeps out to the right. This video explains how to write a parametric equation as an equation in rectangular form. When we parameterize a curve, we are translating a single equation in two variables, such as x and y ,into an equivalent pair of equations in three variables, x, y, and t. X = t2 x = t 2. Here, we have a pair of parametric equations. Consider the path a moon follows as it orbits a planet, which simultaneously rotates around the sun, as seen in (figure). Y = x^2+6x + 9 + 5.
Rectangular Form Of Parametric Equations akrisztina27
Web writing parametric equations in rectangular form Remember, this means we need to rewrite this as an equation in terms of 𝑥 and 𝑦. Web for the following exercises, convert the parametric equations of a curve into rectangular form. Web convert the parametric equations 𝑥 is equal to the cos of 𝑡 and 𝑦 is equal to the sin of.
Rectangular Form Of Parametric Equations akrisztina27
Y = 3x 3 + 5x +6; Web write down the following mentioned rectangular equations into parametric form. Set up the parametric equation for x(t) x ( t) to solve the equation for t t. You have to solve for \(t\) in one of the equations. Let’s evaluate the equation 1:
Rectangular Form Of Parametric Equations akrisztina27
To convert parametric equations to rectangular form, we need to find a way to eliminate the 𝑡. Web how do you convert each parametric equation to rectangular form: Web write down the following mentioned rectangular equations into parametric form. Although we have just shown that there is only one way to interpret a set of parametric equations as a rectangular.
SOLVEDFind a rectangular equation equivalent to the given pair of
Web convert the parametric equations 𝑥 equals 𝑡 squared plus two and 𝑦 equals three 𝑡 minus one to rectangular form. Consider the path a moon follows as it orbits a planet, which simultaneously rotates around the sun, as seen in (figure). This video explains how to write a parametric equation as an equation in rectangular form. X = t2.
Parametric Equations Rectangular Form YouTube
When we parameterize a curve, we are translating a single equation in two variables, such as x and y ,into an equivalent pair of equations in three variables, x, y, and t. Y = (x+3)^2 + 5. Web converting between rectangular and parametric equations. Following steps must be followed in order to convert the equation in parametric form. Web this.
Rectangular Form Of Parametric Equations akrisztina27
F (t)= (x (t),y (t)) given x (t)=t,y (t)=3−t2 select one: Write the parametric equations in rectangular form and identify the interval for x or y line example show more. By convention, t is frequently used as the parameter, though other variables can be used as well. We’re given a pair of parametric equations, and we’re asked to convert this.
Rectangular Form Of Parametric Equations akrisztina27
Set up the parametric equation for x(t) x ( t) to solve the equation for t t. Web converting parametric equation to rectangular form. Web convert x^2 + y^2 = 1 to parametric form. Web parametric equations a rectangular equation, or an equation in rectangular form is an equation composed of variables like x and y which can be graphed.
Rectangular Form Of Parametric Equations akrisztina27
We’re given a pair of parametric equations, and we’re asked to convert this into the rectangular form. Remember, this means we need to rewrite this as an equation in terms of 𝑥 and 𝑦. You have to solve for \(t\) in one of the equations. Y = x 4 + 5x 2 +8; When we parameterize a curve, we are.
How to convert parametric equations to rectangular form example 3 YouTube
Web writing parametric equations in rectangular form Web convert the parametric equations 𝑥 is equal to the cos of 𝑡 and 𝑦 is equal to the sin of 𝑡 to rectangular form. Web write down the following mentioned rectangular equations into parametric form. At any moment, the moon is located at a. Web converting parametric equations to rectangular:
Precalculus Chapter 6.3 Exercises 1118 Convert Parametric Equations
Following steps must be followed in order to convert the equation in parametric form. Rewrite the equation as t2 = x t 2 = x. F (t)= (x (t),y (t)) given x (t)=t,y (t)=3−t2 select one: Web write down the following mentioned rectangular equations into parametric form. Web this is an equation for a parabola in which, in rectangular terms,.
Web Converting Between Rectangular And Parametric Equations.
4.2k views 2 years ago parametric equations. At any moment, the moon is located at a. Y = 3x3 + 5x +6. Web together, x(t) and y(t) are called parametric equations, and generate an ordered pair (x(t), y(t)).
By Convention, T Is Frequently Used As The Parameter, Though Other Variables Can Be Used As Well.
We want to eliminate our. This video explains how to write a parametric equation as an equation in rectangular form. Web write down the following mentioned rectangular equations into parametric form. To convert parametric equations to rectangular form, we need to find a way to eliminate the 𝑡.
Web 1 Day Agoyou'll Get A Detailed Solution From A Subject Matter Expert That Helps You Learn Core Concepts.
Web parametric equations are a set of equations that express a set of quantities as explicit functions of a number of independent variables, known as parameters. for example, while the equation of a circle in cartesian coordinates can be given by r^2=x^2+y^2, one set of parametric equations for the circle are given by x = rcost (1) y. Rewrite the equation as t2 = x t 2 = x. From the curve’s vertex at (1, 2), the graph sweeps out to the right. Web convert x^2 + y^2 = 1 to parametric form.
X = T2 X = T 2.
Web convert the parametric equations 𝑥 equals 𝑡 squared plus two and 𝑦 equals three 𝑡 minus one to rectangular form. To convert this rectangular equation to parametric form, we make use of our knowledge of trigonometry and its identities. Web a typical parametric equation will be in the form x = f ( t) and y = g ( t). Web in the rectangular coordinate system, the rectangular equation y = f ( x) works well for some shapes like a parabola with a vertical axis of symmetry, but in precalculus and the review of conic sections in section 10.0, we encountered several shapes that could not be sketched in this manner.